Projection of Lines - Questions With Answers

 Projection Of Lines

The shortest distance between two points is called a straight line. The projection of two points is a projection of lines. Thus projection of lines is the foundation of engineering drawing.

In this post, we'll practice some questions about the Projection of Lines. Which are as follows:

1. A straight line AB is 60 mm long. It is inclined to H.P. and V.P. by an angle of 30° and 45° respectively. Point A is 30 mm above H.P. and 20 mm in front of V.P. Draw the projections of straight line AB. Find also the traces.
2. The elevation of line AB, 80 mm long, measures 55 mm. The end A is 20 mm above H.P. and 10 mm in front of V.P. Draw the projections of the line and find its true inclinations with H.P. and V.P. If the end B is 25 mm below H.P. and is behind V.P.
3. Find M of a line MN, which is inclined at 46° to H.P. and 20° to V.P. is 15 mm above the H.P. and it is in front of the V.P., while the end N is 60 mm in front of V.P. and is above H.P. Draw the projections of the line, find its true length if its plan length is 70 mm. Locate the points of intersection of the line with the principal planes.

If you want to solve all the problems on your own, It is best for you. In case you are unable to solve it, don't worry we'll see the solution to these questions one by one. We are going to start with the easiest question.

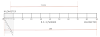
(1) Question: A straight line AB is 60 mm long. It is inclined to H.P. and V.P. by an angle of 30° and 45° respectively. Point A is 30 mm above H.P. and 20 mm in front of V.P. Draw the projections of straight line AB. Find also the traces.

Given data:

• TL = 60 mm
• θ = 30°
• ɸ = 45°
• a' = 30 mm above HP
• a = 20 mm in front of the VP

Solution:

Procedure:

• Draw an XY line and a vertical line as shown in the figure.
• Project point A according to the given data. (If you don't know the concept of projections of points, click here to learn: Projection of Points)
• The line is inclined to HP by 30° so draw a line from point a' of the length of 60 mm (True length). Name the other endpoint of the line as b1'.
• Now because the line is inclined to VP by 45°, draw a line from the point a of 60 mm length. Name the other end of the line as b1. 
• Draw a vertical line from point b1' to the locus of a as shown in the figure. Name that point g.
• Take a compass with a radius of ag and draw an arc to the locus of b.
• At where the arc and locus of b intersect, name that point b.
• Draw the line ab which will be the top view of the line. 
• Measure its length( should be around 53 mm) and inclination( should be around 56°) from the locus of a.
• For the front view of the line, simply draw a vertical line from point b to the locus of b'.
• Where the vertical line and the locus of b' intersect, name that point b'.
• Draw the line a'b' which will be the front view of the line.
• Measure its length( should be around 43 mm) and inclination( should be around 45°) from the locus of a'.
• For the trace, expand the lines ab and a'b' to the locus of a and XY line respectively another side of the vertical line.
• Where the lines intersect with XY line and name them v and h'.
• Draw vertical lines from points v and h'.
• Where the lines intersect with those vertical lines name those points h'T and vT as shown in the figure.
• Your problem is solved.

I hope you understood the question and solution as well. Now let's move forward to the 2nd question, which is as follows:

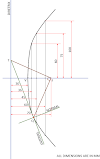
(2) Question: The elevation of line AB, 80 mm long, measures 55 mm. The end A is 20 mm above H.P. and 10 mm in front of V.P. Draw the projections of the line and find its true inclinations with H.P. and V.P. If the end B is 25 mm below H.P. and is behind V.P.

Given data:

• TL = 80 mm
•  FVL = 55 mm
• a' = 20 mm above HP
• a = 10 mm in front of the VP
• b' = 25 mm below HP

Solution:

Procedure:

• Project the given points (a' and a) according to the question and draw the respective locus of the points.
• To project the point b', we have to draw a horizontal line (which will be the locus of b') 25 mm below the X-Y line. With the help of FVL (Front View Length) given in the question, we can project point b'.
• Take a compass and measure 55 mm(= 5.5 cm) with the help of a scale. Now taking the center as the point a' draw an arc on the locus of b'. Which will be the point b'.
• Connect the points a' and b' which is the front view of the line AB.
• Now, for the true length, take a compass and measure 80 mm(= 8 cm) with the help of a scale. Now taking the center as the point a' draw an arc on the locus of b'. Which will be the point b₁'.
• Connect the points a' and b₁' which is the true length of the line AB.
• Now, to draw the top view of line AB draw a vertical line from point b₁' to the locus of a. Where the vertical line and locus of a intersects, name that point as g.
• Now, take a compass and measure the distance ag and taking center as the point a, draw an arc from the point g to the upper side of the line X-Y (As long as it seems to be required according to you). 
• Draw a vertical line from the point b' to the arc which you drew in the previous step. Where they intersect, name that point b and draw the locus of b.
• Connect points a and b which is the top view of line AB.
• Now, for the true length, take a compass and measure 80 mm(= 8 cm) with the help of a scale. Now take the center as point a and draw an arc on the locus of b. Which will be the point b₁.
• Connect the points a and b₁ which is the true length of the line AB.
• At last, measure all the lengths and angles of the lines which should be equal to the given below: 

• 𝛂 = 55°
• 𝛃 = 62°
• 𝝷 = 35°
• 𝞍 = 46°
• TVL = 65 mm

I hope you understood the question and solution as well. Now let's move forward to our last question, which is as follows:

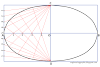
(3) Question: Find M of a line MN, which is inclined at 46° to H.P. and 20° to V.P. is 15 mm above the H.P. and it is in front of the V.P., while the end N is 60 mm in front of V.P. and is above H.P. Draw the projections of the line, find its true length if its plan length is 70 mm. Locate the points of intersection of the line with the principal planes.

Given Data:

• θ = 46°
• ɸ = 20°
• m' = 15 mm above HP
• n = 60 mm in front of VP
• TVL = 70 mm

Solution: 

Procedure:

• Project the point m' according to the question and draw the locus of n 60 mm (= 6 cm) below the XY line.
• Draw a vertical line at the distance of 70 mm (= 7 cm) from the point m' (Because TVL (Top View Length) is 70 mm (= 7 cm)).
• Now for the true length, draw a line at the inclination of 46° from the point m'. Where the vertical line and the inclined line intersect, name that point n₁'. Also, draw the locus of n'.
• Connect the points m' and n₁' which is the true length of the line MN. Measure the length of the line which should be equal to 100 mm (= 10 cm).
• Now, to find the position of the point m, draw a line at the inclination of 20° at XY line as shown in the solution.
• Take a roller scale and put it parallel to the inclined line which you drew in the last step.
• Roll down the scale until the distance between the locus of n and the vertical line (in green color shown in the solution) becomes exactly 100 mm (= 10 cm).
• Draw a line at that position of 100 mm (= 10 cm) which is the true length of the line MN.  
• Where that line and vertical line (in green color shown in the solution) intersect, name that point m. Also, draw the locus of m.
• To make the top view of the line MN, draw an arc of 70 mm taking the center as the point m, to the locus of n.
• Where they intersect, name that point n and connect the points m and n. Which is the top view of the line MN.
• To draw the front view, draw a vertical line from the point n to the locus of n'. Where they intersect, name that point n'.
• Connect the points m' and n' which is the front view of the line MN.
• Show all the necessary dimensions as shown in the solution.

Asked Data:

• TL = 100 mm
• m = 25 mm in front of the VP

I hope you understood all the questions and solutions very well. If you have any doubts regarding these solutions or questions, let me know in the comment section. Till then keep learning, and keep improving!